From geometric equations to dynamic strategies: advances in the personalization of mechanical ventilation through mechanical power

González-Castro, Alejandro; Fajardo Campoverdi, Aurio

doi:10.1016/j.medine.2024.11.004

ISSN: 2173-5727

Medicina Intensiva is the journal of the Spanish Society of Intensive and Critical Care Medicine and Coronary Units (SEMICYUC), and has become the reference publication in Spanish in its field. Medicina Intensiva mainly publishes Original Articles, Reviews, Clinical Notes, Images in Intensive Medicine, and Information relevant to the specialty. All works go through a rigorous selection process.

Indexed in:

Index Medicus / MEDLINE / EMBASE / Excerpta Medica / SCOPUS / MEDES / Science Citation Index Expanded, Journal of Citation Reports

Subscribe:

Impact factor

The Impact Factor measures the average number of citations received in a particular year by papers published in the journal during the two preceding years.

© Clarivate Analytics, Journal Citation Reports 2022

Impact factor 2023

2.7 (Q2)

Citescore

CiteScore measures average citations received per document published.

Citescore 2023

2.7

SJR

SRJ is a prestige metric based on the idea that not all citations are the same. SJR uses a similar algorithm as the Google page rank; it provides a quantitative and qualitative measure of the journal's impact.

SJR 2023

0.341

SNIP

SNIP measures contextual citation impact by wighting citations based on the total number of citations in a subject field.

SNIP 2023

0.57

View more metrics

Open Access option

Journal Information

Previous article | Next article

Export reference

Download PDF

More article options

Statistics

Letter to the Editor

DOI: 10.1016/j.medine.2024.11.004

Available online 20 November 2024

From geometric equations to dynamic strategies: advances in the personalization of mechanical ventilation through mechanical power

De ecuaciones geométricas a estrategias dinámicas: avances en la personalización de la ventilación mecánica mediante la potencia mecánica

Visits

Download PDF

Alejandro González-Castroa,c,

Corresponding author

e409@humv.es

Corresponding author.

, Aurio Fajardo Campoverdib,c

a Servicio de Medicina Intensiva, Hospital Universitario Marqués de Valdecilla, Spain

b Critical Care Unit, Hospital Biprovincial Quillota-Petorca, Chile

c Grupo Internacional de Ventilación Mecánica, WeVent, Spain

This item has received

1 Visits

Article information

Full Text

Bibliography

Download PDF

Statistics

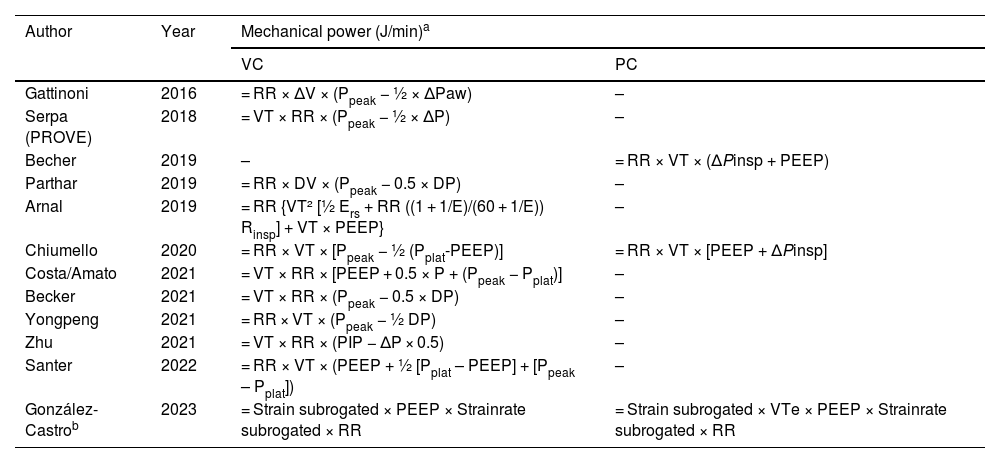
Tables (1)

Table 1. Main equations proposed since 2016 to estimate mechanical power in both volume and pressure control.

Article

These are the options to access the full texts of the publication Medicina Intensiva (English Edition)

Member of the society

Subscriber

Contact

Member

If you are a member of the Sociedad Española de Medicina Intensiva, Crítica y Unidades Coronarias::
Go to the members area of the website of the SEMICYUC (www.semicyuc.org )and click the link to the magazine.

Subscriber

If you already have your login data, please click here .

If you have forgotten your password you can you can recover it by clicking here and selecting the option “I have forgotten my password”

Subscribe to

Medicina Intensiva (English Edition)

More information

Purchase

Purchase article

Purchasing article the PDF version will be downloaded

Price 19.34 €

Purchase now

Contact

Phone for subscriptions and reporting of errors

From Monday to Friday from 9 a.m. to 6 p.m. (GMT + 1) except for the months of July and August which will be from 9 a.m. to 3 p.m.

Calls from Spain

932 415 960

Calls from outside Spain

+34 932 415 960

atencionalcliente@elsevier.com

Indexed in:

Follow us:

Subscribe:

Article

Subscribe to our newsletter